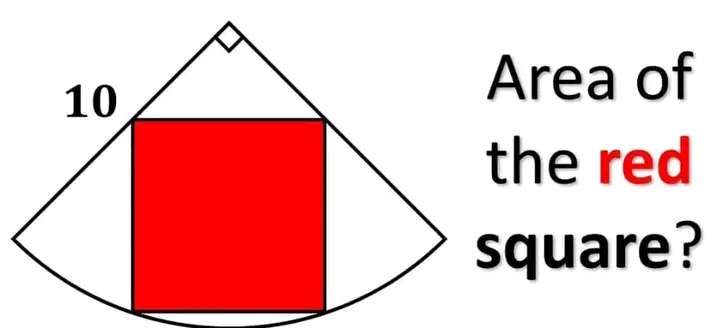
Let the side length of the inscribed square be a.
Calculating the area of the inscribed red square.
2b² = a²
b = ½√(2)a unit.
c² = 2a²
c = √(2)a unit.
c is the diagonal of the inscribed...
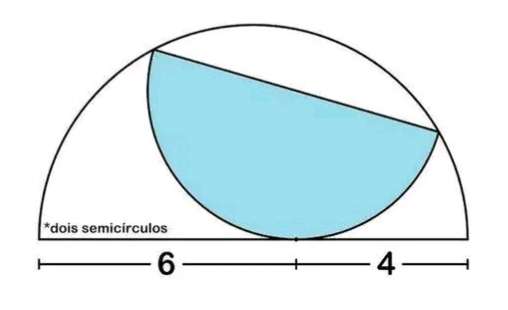
Calculating area of the inscribed blue half circle.
a = ½(6+4)
a = 5 units.
a is the radius of the ascribed semi circle.
b = a-4
b = 1 unit.
c²+1² = d²
d = √(c²+1) units.
c is the rad...

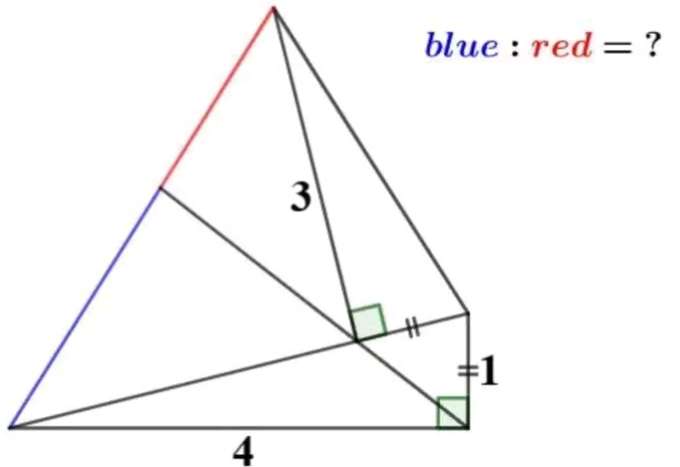
Notice!
Triangle ABC is equilateral.
Let AB be 1 unit.
a = 180-42-12
a = 126°
a is angle BDC.
(1/sin126) = (b/sin42)
b = 0.8270909153 units.
b is CD.
c² = 1+0.8270909153²-2*0.827...
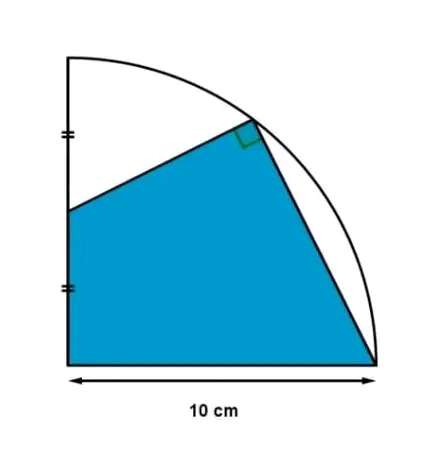
a² = 4²+1²
a = √(17) units.
b = a-1
b = (√(17)-1) units.
b = 3.1231056256 units.
c² = (√(17)-1)²+3²
c² = 17-2√(17)+1+9
c = √(27-2√(17)) units.
c = 4.3305644838 units.
tand = (√(17)-1...
a = 10+10
a = 20 cm.
a is twice the radius of the quarter circle.
b = ½(10)
b = 5 cm.
c² = 5²+10²
c √(125)
c = 5√(5) cm.
Observing similar plane shape (right-angled triangle) side len...

Calculating area of the blue a.
b² = 2a²
b = √(2)a cm.
b is the diagonal of the blue square.
c = 7-5
c = 2 cm.
d = 7+5
d = 12 units.
It implies;
(√(2)a)² = 2²+12²
2a² = 148
a²...
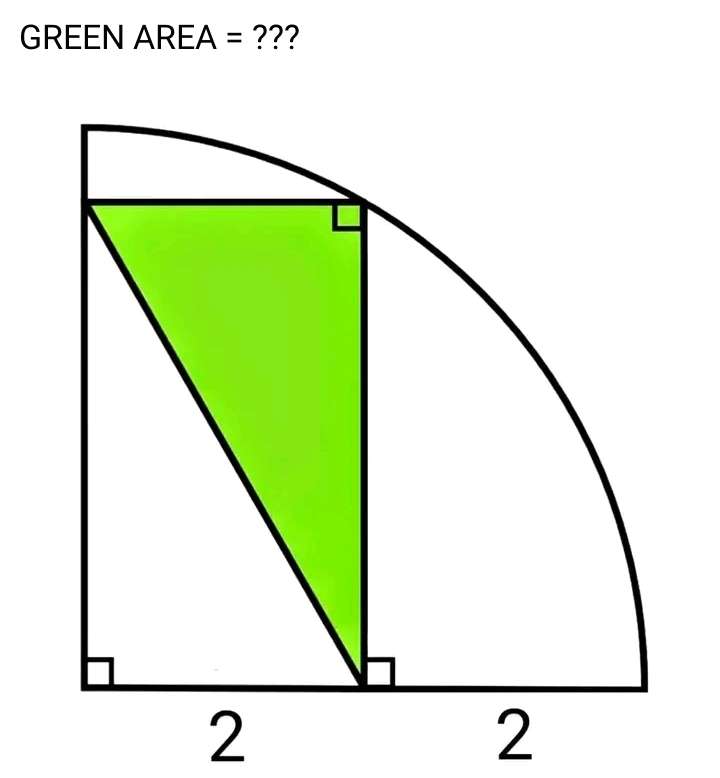
a = 2+2
a = 4 units.
a is the radius of the quarter circle.
b² = 4²-2²
b² = 16-4
b = √(12)
b = 2√(3) units.
Therefore;
Area triangle green is;
½*2√(3)*2
= 2√(3) square units.
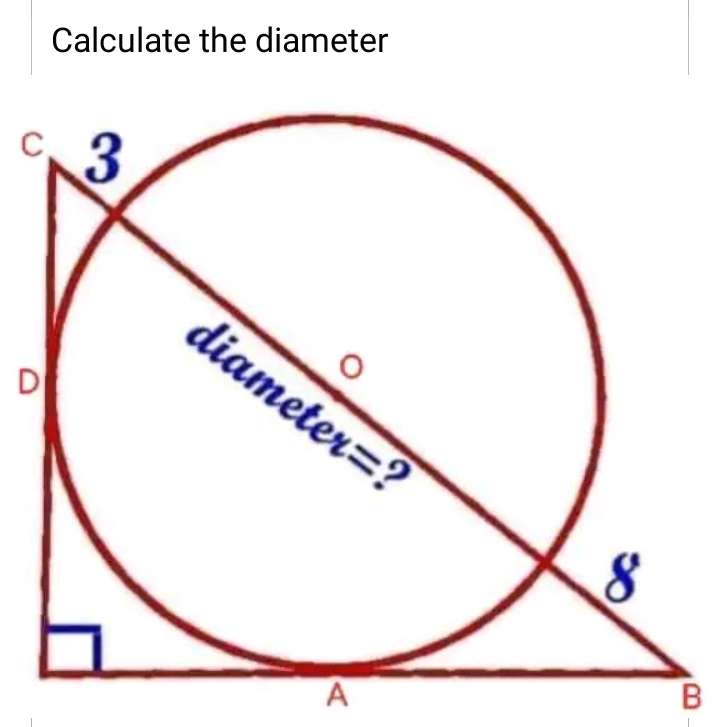
Let the radius of the circle be a.
b = (3+a) units.
c = (8+a) units.
d² = (3+a)²-a²
d = √(9+6a) units.
Observing similar plane shape (right-angled) side length ratios.
a - √(9+6a)...
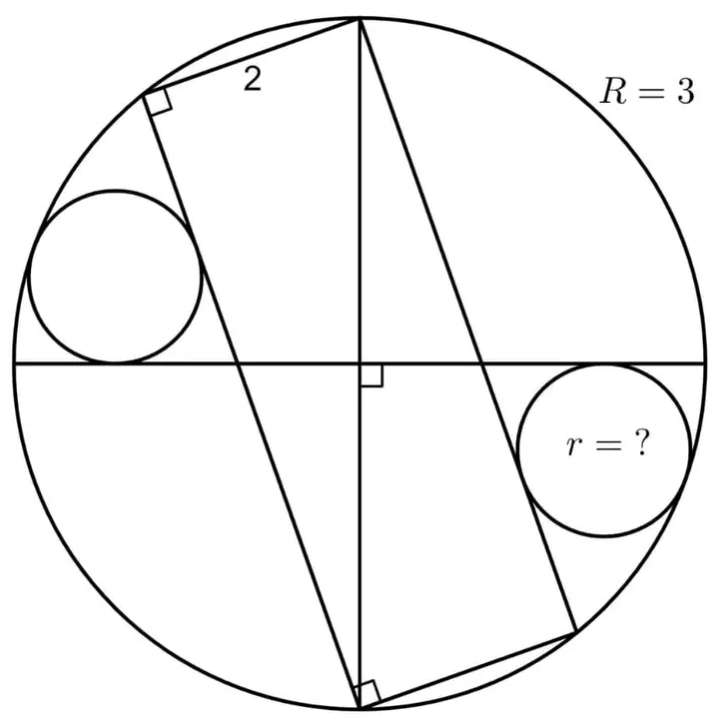
Diameter of the ascribed circle is;
2R
= 2*3
= 6 units.
a²+2² = 6²
a = √(32)
a = 4√(2) units.
Observing similar plane shape (right-angled) side length ratios.
3 - 4√(2)
b - 2
Cros...
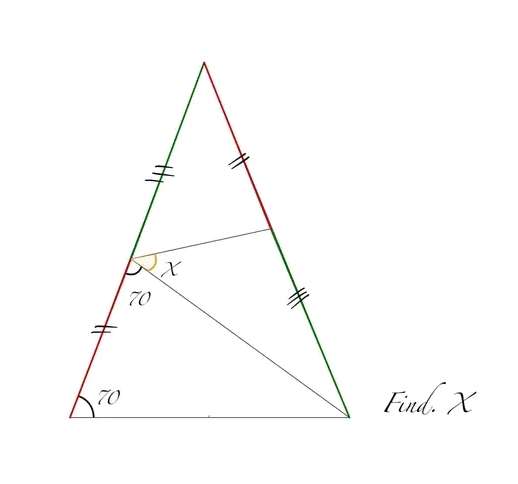
Let the base of the ascribed triangle be 1 unit.
a = 180-70-70
a = 40°
(b/sin40) = (1/sin70)
b = 0.6840402867 units.
Considering similar plane shape (isosceles triangle) side length rati...