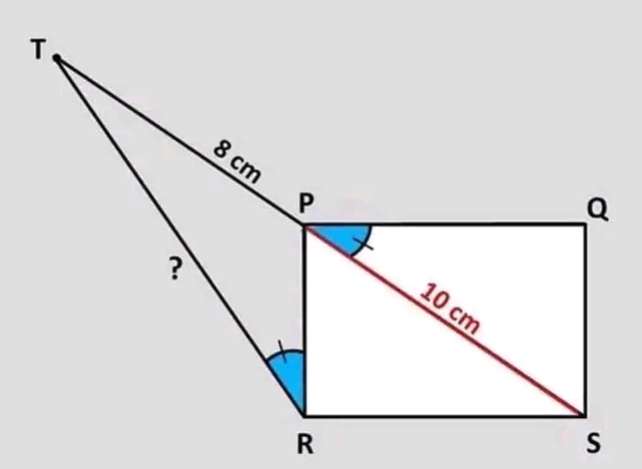
RT = √((8+5)²-5²)
RT = √(169-25)
RT = √(144)
RT = 12 cm.
RT is the required length.
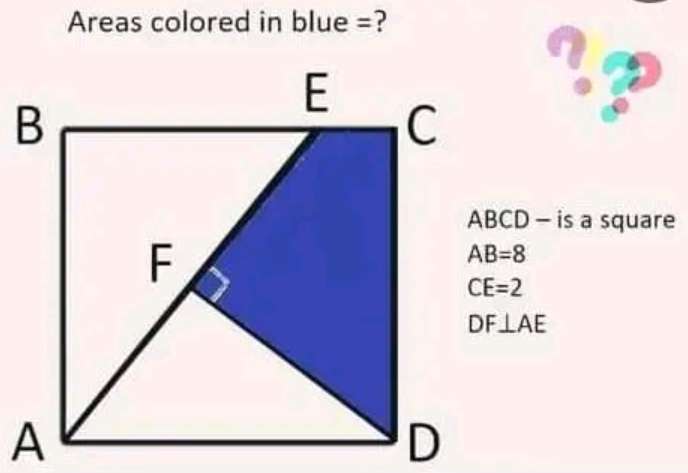
Blue area will be;
½(5.2x6.4)+½(2x8)
= ½(33.28)+8
= 16.64+8.00
= 26.64 square unit.
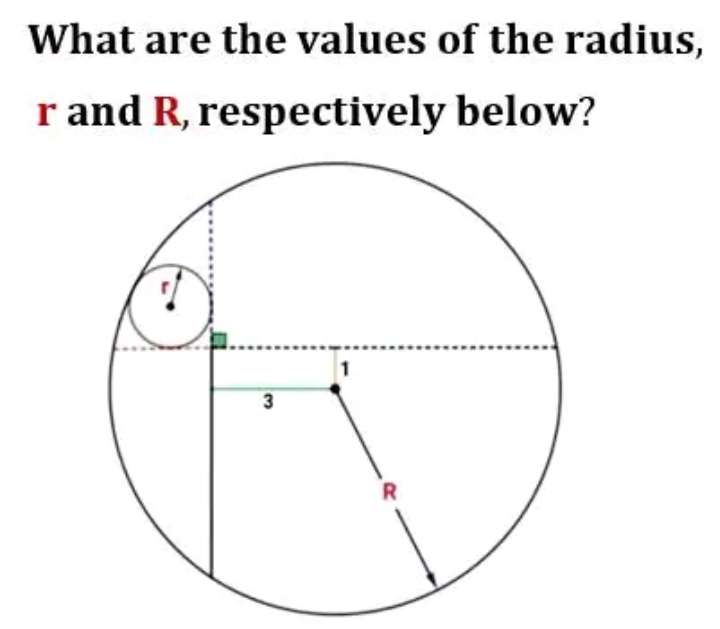
Calculating R.
a²+3² = R²
a = √(R²-9) units.
b = 1+a
b = (1+√(R²-9)) units.
c = a-1
c = (√(R²-9)-1) units.
d²+1² = R²
d = √(R²-1) units.
e = 3+d
e = (3+√(R²-1)) units.
f = d-...
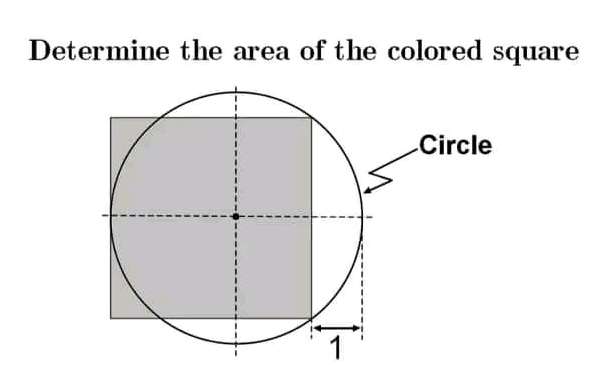
Let r be the radius of the circle.
a = (r-1) units.
b = (r-0.5) units.
Calculating r.
It implies;
r² = (r-1)²+(r-0.5)²
r² = r²-2r+1+r²-r+(¼)
0 = r²-3r+(5/4)
4r²-12r+5 = 0
4r²-2r-...

Let a be 1 units.
a is the side length of the regular hexagon.
Calculating b.
c = 120-90
c = 30°
120° is the single interior angle of the regular hexagon.
cos30 = 1/d
d = 2/√(3)
d = ⅓(2√(3)) unit...
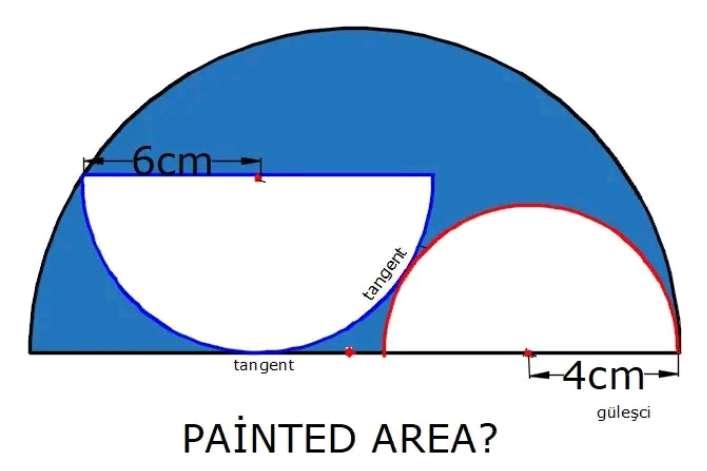
a = 6+4
a = 10 cm.
b²+6² = 10²
b = √(100-36)
b = √(64)
b = 8 cm.
c = b+4
c = 12 cm.
d = c-r
d = (12-r) cm.
r is the ascribed half circle radius.
e = 6+d
e = 6+(12-r)
e = (18-r)...
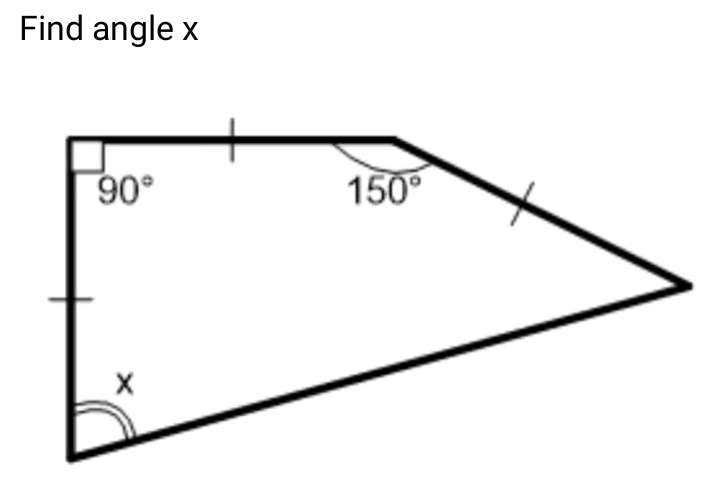
Let the three equal lengths be 1 unit each.
a² = 1²+1²
a = √(2) units.
b = 150-45
b = 105°
c² = √(2)²+1²+2√(2)cos 105
c = 1.9318516526 units.
(1.9318516526/sin105) = (1/sind)
d = 30...

Let AD be 4 units.
Therefore;
AB will be;
½(AD)+½(½(AD))
AB = ½(4)+½(½(4))
AB = 2+1
AB = 3 units.
Area ABCD is;
4*3
= 12 square units.
Calculating Area Red (Area Trapezoid)....
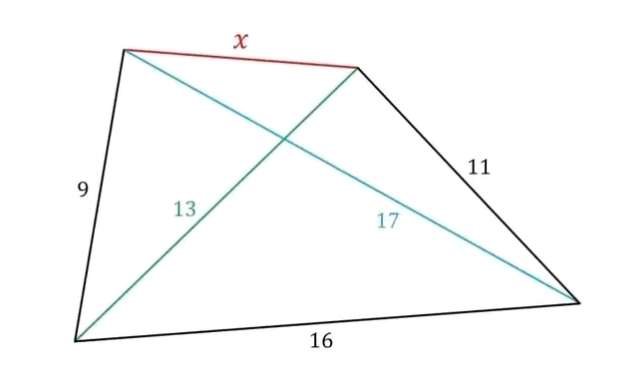
9² = 16²+17²-2*16*17cosa
81 = 545-544cosa
544cosa = 545-81
cosa = 464/544
a = 31.4669762933°
13² = 16²+11²-2*16*11cosb
169 = 377-352cosb
352cosb = 377-169
cosb = (208/352)
b = 53.7784533...
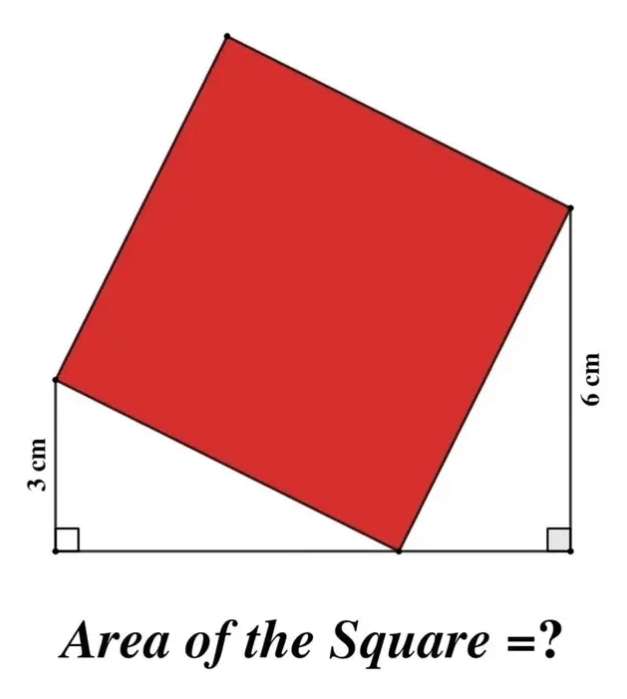
Let the side length of the red square be a units.
b²+3² = a²
b = √(a²-9) units.
c²+6² = a²
c = √(a²-36) units.
Observing similar plane shape (right-angled) side length ratios.
√(a²-9)...