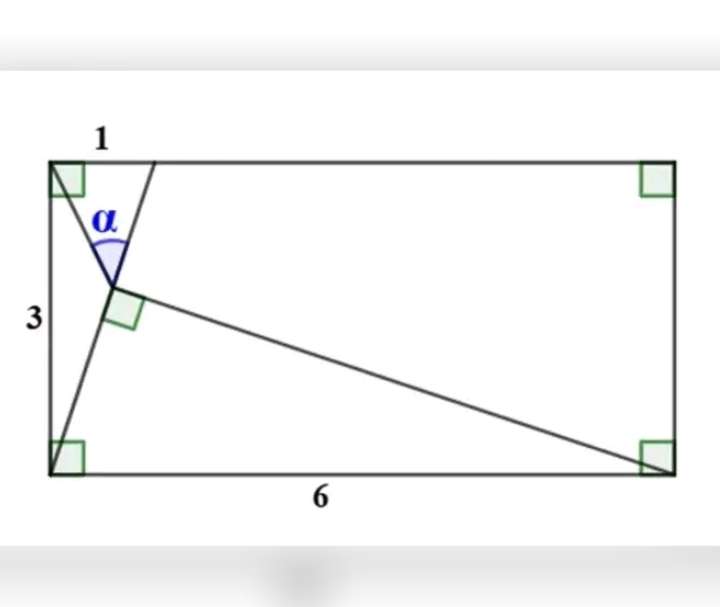
tana = 1/3
a = atan(⅓)°
b = 90-a
b = atan(3)°
cos(atan(3)) = c/6
c = 1.8973665961 units.
d² = 1.8973665961²+3²-2*3*1.8973665961*(1/3)
d = 1.3416407865 units.
(1.3416407865/sin(atan(...
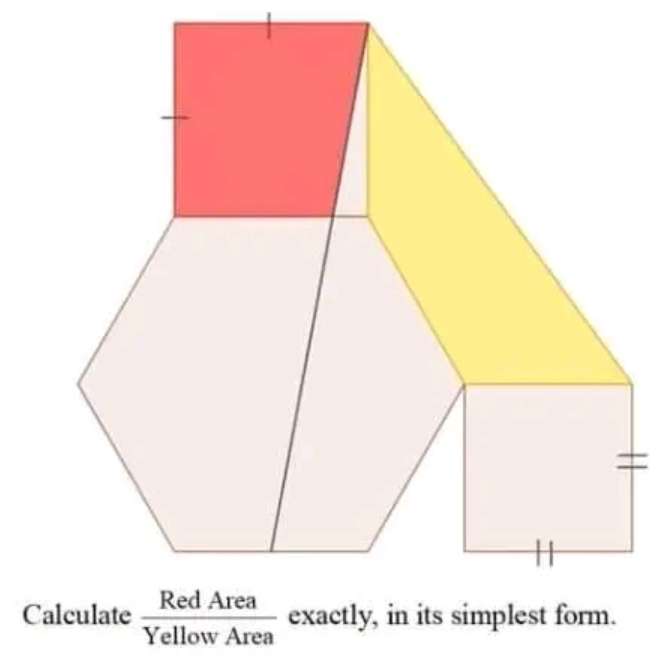
Let the single side length of the regular hexagon be 2 unit.
Therefore;
Area Red is;
4 - ½*½(2/(√(3)+1))
= 4 - ((√(3)-1)/2)
= ½(9-√(3)) square unit.
Area Yellow is;
½(√(3)+2)(√(3)+...
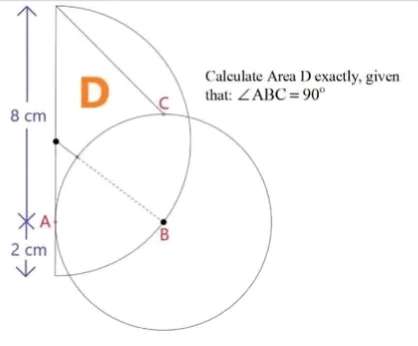
Sir Mike Ambrose is the author of the question.
Area D exactly in cm² is;
Area trapezoid with parallel sides 4 cm and 8 cm, and height 4 cm - Area triangle with height 4 cm and base 3 cm - Ar...
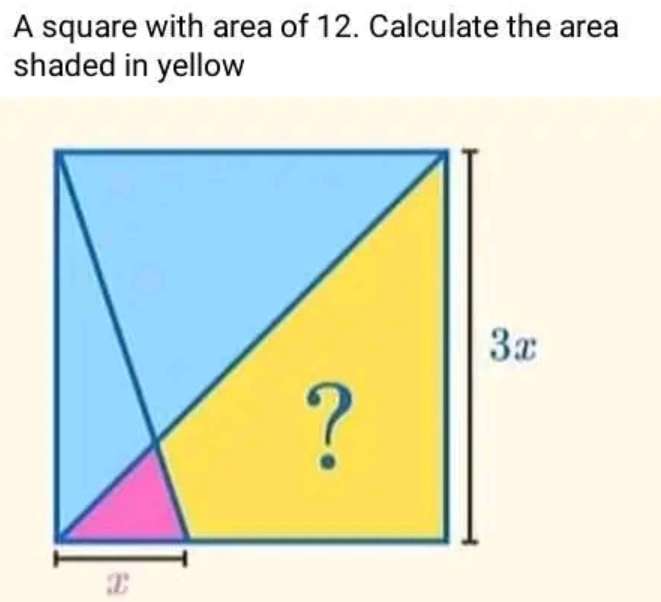
Yellow area will be;
½(12) - (½(⅔√(3))²xsin(atan(3))÷sin(135-atan(3)))xsin(45)
= 6 - (½)
= ½(12-1)
= ½(11)
= 5.5 square unit.

Area red is;
½(3*4) - (atan(4/3)x9π÷ 360) - (atan(3/4)x4π÷ 360)
= 0.54016930141 square units.

Calculating Angle x.
Let the two equal lengths of the ascribed triangle be 1 unit each.
a = 180-40-30
a = 110°
b = 180-a
b = 70°
(1/sin40) = (c/sin30)
c = 0.7778619134 units.
Ther...
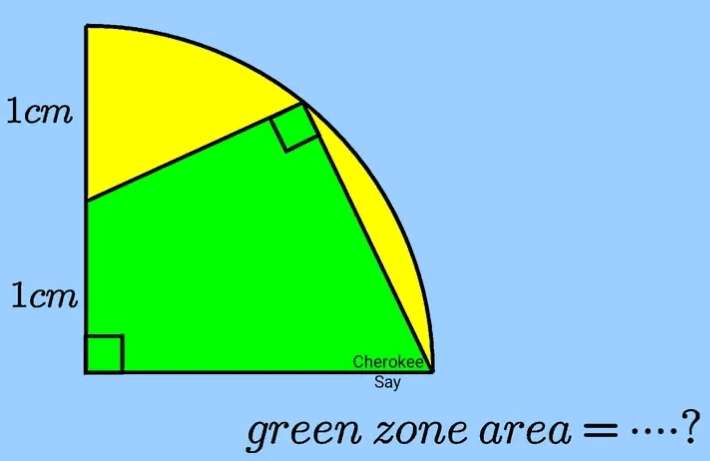
Radius of the ascribed quarter circle is;
1+1 = 2 cm.
a² = 1²+2²
a = √(5) cm.
Observing similar plane shape (right-angled) side length ratios.
√(5) - 4
1 - b
Cross Multiply.
b =...
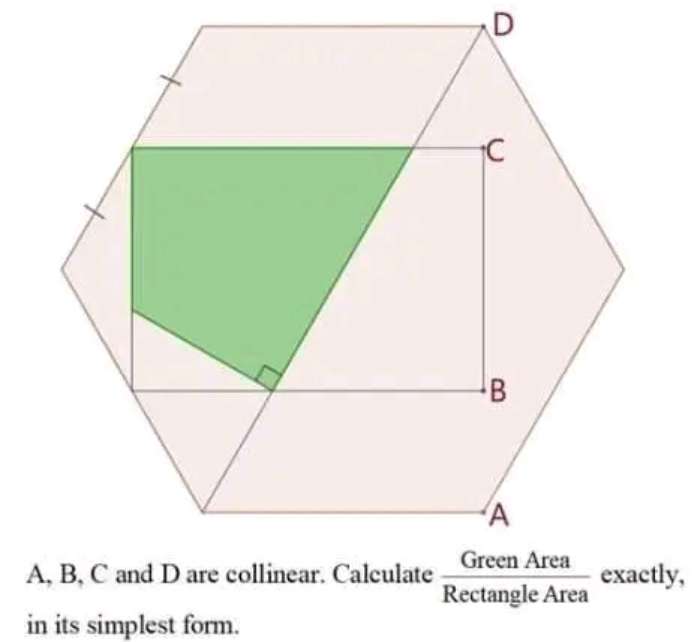
Sir Mike Ambrose is the author of the question.
Let the single side length of the regular hexagon be 2 unit.
Area rectangle is;
2.5x√(3)
= ½(5√(3)) square units.
Area green is;
Area t...
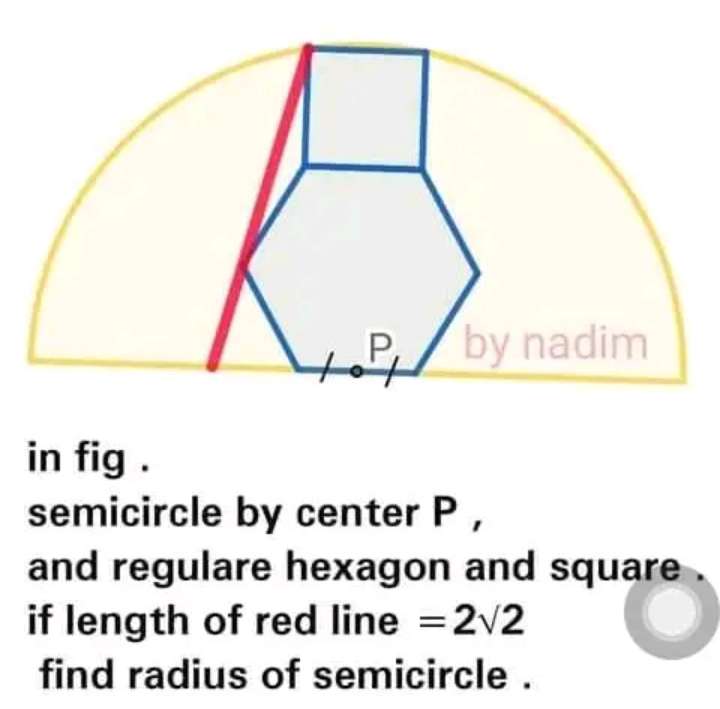
Let the radius of the semicircle be r.
Therefore;
r²= (1+√(3))²+(½)²
r²=1+2√(3)+3+¼
r²=4+¼+2√(3)
r²=¼(17+8√(3)
r = ½√(17+8√(3)) units.
r = 2.77742715749 units.
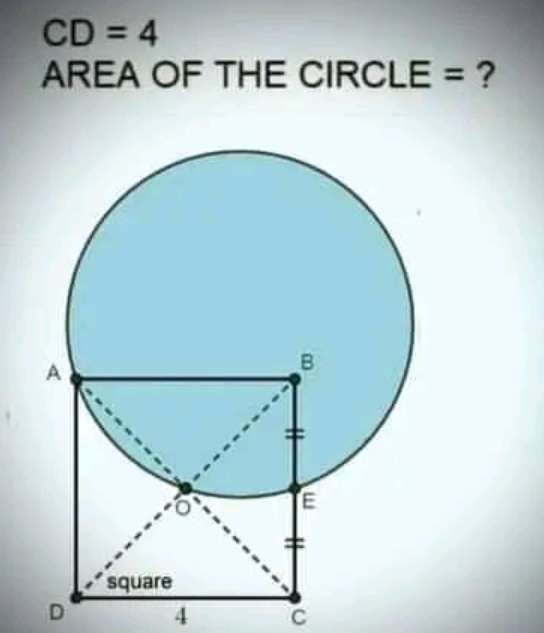
Let the radius of the circle be r.
Therefore;
(2r)²=2(√(20))²
4r²=40
r = √(10) unit.
Therefore area of the circle is;
πr²
π(√(10))²
= 10π square unit.