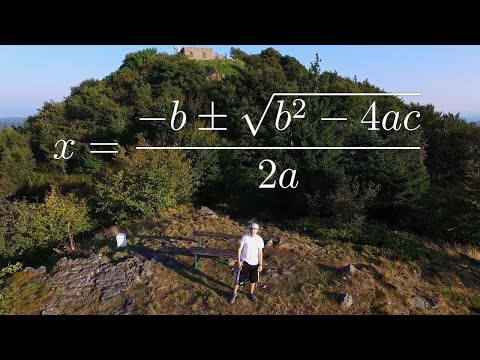“Mathematics is the most beautiful and most powerful creation of the human spirit.”

Stefan Banach

“To not know math is a severe limitation to understanding the world.” Richard Feynman

Math solutions and review, a good medication for the brain.

“The study of mathematics, like the Nile, begins in minuteness but ends in magnificence.”

Charles Caleb Colton

Super Smart and Amazingly, Very Clever!

We are smart and clever!

The important thing to remember about Mathematics is not to be frightened.

Enquire

Announcements

OnlineEdumath

Year of Establishment
March, 2020

6
Experienced Faculty

We mentor/educate/teach learners Mathematics online via Google Meet App. Our Certified, Resilient and Productive Mathematics Educators make teaching and learning Mathematics fun for learners. Communicate us to mentor your child/children to become a lover of Mathematics, and a Mathematician

Call Us Mail Us Reach Us

Our Updates

We are smart and clever!

post

We are smart and clever!

By OnlineEdumath | 5th November, 2024

“Mathematics is the most beautiful and most powerful creation of the human spirit.” Stefan Banach

Welcome to Online Edumath

post

Welcome to Online Edumath

By OnlineEdumath | 4th September, 2024

This is Online Edumath website created by Ogheneovo Daniel Ephivbotor. Online Edumath falls in EDUCATION line of business. You can visit us offline at our office located at : Lugbe, Federal...

We are smart and clever!

post

We are smart and clever!

By OnlineEdumath | 3rd July, 2024

For enquiry on what we do and on our shared Mathematics Questions and Solution review, please communicate us via our WhatsApp contact: +2349076614992 We are kindly to respond to your question(s)/...

Thank You, Sir Bill.

post

Thank You, Sir Bill.

By OnlineEdumath | 18th April, 2024

The Management and Educators of Online Edumath kindly appreciate your words of affirmation and encouragement, Sir Bill. We are grateful! We pledge to keep working hard as we mentor/educate our lea...

We are smart and clever!

post

We are smart and clever!

By OnlineEdumath | 18th April, 2024

Online Edumath Educators and Learners are Super Smart and Amazingly, Very Clever. Communicate us to mentor/teach/educate your child/children Mathematics online at affordable tuition, helping them be...

Mr. Daniel (Mathematics Educator)

post

Mr. Daniel (Mathematics Educator)

By OnlineEdumath | 26th August, 2023

Mr. Daniel is one of our Educators, He is a certified, hardworking, dedicated and passionate Mathematics Educator that aim at taking the sincere, profound knowledge of Mathematics to the comfort of...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 2nd August, 2025

Calculating Area Triangle ABC and Length AN ÷ Length NC. Let AB equal x. a = (3*x)/(3+4) a = ⅐(3x) units. a is AT = AN. b = (4*x)/(3+4) b = ⅐(4x) units. b is TB = MB. c = a+4 c = ⅐(3x)+4 c = ⅐(3...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 2nd August, 2025

Calculating Blue Area. Blue Area is; 2(area circle of radius 1 unit - 2(2(area sector of radius 1 unit and angle 60°) - area equilateral triangle of two side length 1 unit and angle 60°)) =...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 2nd August, 2025

Sir Mike Ambrose is the author of the question. Calculating Area Green ÷ Area Rectangle. Area rectangle is; 9x8 = 72 square units. Area green is; Area trapezoid with two parallel lengths (5+2-¾)...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 1st August, 2025

Calculating Blue Area. Let x be the radius of the ascribed half circle. a²+7² = (2x)² a = √(4x²-49) units. b = a-4 b = (√(4x²-49)-4) units. It implies; Calculating x. √(4x²-49) ~ x 2x ~ (√(4x²...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 1st August, 2025

Calculating Area Inscribed Yellow Square. Let the inscribed yellow square side length be x. Calculating x. a = (8-x) units. b = (8-2x) units. It implies; 8² = (8-2x)²+(8-x)² 64 =...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 1st August, 2025

Calculating Green Area. Notice. The composite plane shape is not drawn to scale. Let x be the width of the ascribed rectangle. Therefore; x = the diameter of the inscribed small half circle, an...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 31st July, 2025

Calculating Area Yellow. Let y be each of the two equal lengths that makes the length of the ascribed rectangle. Calculating Yellow Area. a = 14+18 a = 32 units. a is the width of the ascr...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 31st July, 2025

Sir Mike Ambrose is the author of the question. Let the two equal length of the inscribed isosceles triangle (red area) be 2 units. Therefore; Area blue will be; Area triangle with lengths 1 unit...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 30th July, 2025

Calculating square area. tana = (k+2k)/k a = atan(3)° b = 90-a b = atan(⅓)° tanc = 3k/2k c = atan(3/2)° d = 180-a-c d = 180-atan(3)-atan(3/2) d = 52.1250163489° e = 180-a e = (9...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 30th July, 2025

Calculating Blue Inscribed Square Area. Let a be the side length of the inscribed blue square. b = a+½(a) b = ½(3a) units. 6² = 2c² c² = ½(36) c = √(18) c = 3√(2) units. Notice....

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 30th July, 2025

Calculating Shaded Area. Notice. The ascribed square side length is 1 unit. tana = 1/½ a = atan(2)° b = 2a b = 2atan(2)° c = b-90 c = 2atan(2)-90 c = 36.8698976458° d = 180-b d = 180-2atan(2)...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 29th July, 2025

Calculating Blue Shaded Area. Let a be the radius of each of the circle with area 10 square units. Calculating a. πa² = 10 a² = 10/π a = √(10/π) units. a = 1.78412411615 units. Area...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 29th July, 2025

Calculating r, radius of the inscribed semicircle. 15² = 13²+14²-2*13*14cosa 2*13*14cosa = 13²+14²-15² cosa = (13²+14²-15²)/(2*13*13) a = 67.380135052° It implies, r is; 13r+14r = 13*14sin67.3801...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 28th July, 2025

Calculating Area Square. Let x be the side length of the square. a²+x² = 3² a = √(9-x²) units. b = x-a b = (x-√(9-x²)) units. Therefore; x/3 = (x-√(9-x²))/2 Cross Multiply. 2x =...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 28th July, 2025

Calculating Area Blue. Let a be the side length of the ascribed quarter circle. tan30 = 2/b b = 2√(3) units. c = (a-2) units. d = (2√(3)+½(a)) d = ½(4√(3)+a) units. Calculating a. (a-2)² = (½(...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 27th July, 2025

Calculating Area Yellow, Area of The Inscribed Half Circle. 4r+3r = 4*3 7r = 12 r = ⅐(12) units. r is the radius of the inscribed yellow half circle. Area Yellow Inscribed Half Circle is;...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 27th July, 2025

Calculating Area Green. sin30 = a/12 a = 6 units. b²+6² = 12². b = √(144-36) b √(108) b = 6√(3) units. sin60 = c/12 c = 6√(3) units. d² = 12²-(6√(3))² d = √(144-108) d = √(36) d = 6 units. The...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 26th July, 2025

Calculating Shaded Green Area. sin30 = a/2 a = 1 unit. (a')²+1² = 2² a' = √(4-1) a' = √(3) units. a'' = 2a' a'' = 2√(3) units. a'' is the side length of the inscribed regular triangle. b² = 2²+2...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 26th July, 2025

Calculating Length x. x ~ ½(2) (2+7) ~ x Cross Multiply. x² = 9 x = √(9) x = 3 units.

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 26th July, 2025

Calculating Area Blue Triangle. a² = 2(2)² a = 2√(2) units. a is the diagonal of a square. b = ½(180-30) b = 75° c = b-45 c = 75-45 c = 30° sin30 = d/a ½ = d/(2√(2)) d = √(2) units. d is the bas...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 25th July, 2025

Calculating r/R. Let let the ascribed square side length be 2 units. Calculating R. a = ½(2) a = 1 unit. b = (2-R) units. Therefore; (2-R)² = R²+1 4-4R+R² = R²+1 4-1 = 4R R = ¾...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 25th July, 2025

Calculating Shaded Fraction. Let the ascribed square side length be 5 units. a = 1 unit. a is the side length of the big inscribed yellow square. b = ½(5-a) b = ½(5-1) b = 2 units. b is the side l...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 24th July, 2025

Calculating the required angle. Let it be x. a = ⅑*180(9-2) a = 20*7 a = 140° a is the single interior angle of the regular nonagon. b = ½(140)-65 b = 5° c = ½(180(5-2)-3(140)) c = ½(540-420) c...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 24th July, 2025

Calculating Length x. Let a be the side length of the blue square. b² = 2a² b = √(2)a units. b is the diagonal of the blue square. It implies; x ~ 3 √(2)a ~ a Cross Multiply. a...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 24th July, 2025

Calculating Red Area. Let AB = CD = x. Let AD = BC = y. ½*xa = 10 a = 20/x units. a is BQ. ½*xb = 6 b = 12/x units. b is the horizontal height of area 6 (area triangle APB). c = y-b c = y-(12/x...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 23rd July, 2025

Calculating area ABCD. Let x be the radius of the inscribed circle. a = ½(16) a = 8 units. x² = 8²+a² a = √(x²-64) units. b = ½(25) units. It implies; x ~ 8 ½(25) ~ x Cross Multiply. x² = 10...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 23rd July, 2025

Calculating Area Yellow. ½*a²sin60 = 24 √(3)a² = 24*4 a² = 96/√(3) a = √(96/√(3)) a = 7.44483887282 units. a is the radius of the circle. b = a-x b = (7.44483887282-x) units. Calcul...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 23rd July, 2025

Sir Mike Ambrose is the author of the question. Let the single side length of the square be 2 units. Area square is; 2² = 4 square units. Calculating green area. It will be; Area square of side...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 22nd July, 2025

Let the single side length of the ascribed square be 2 units. Calculating yellow area . It is; Area sector with radius 2 units and angle (90-2tan–¹(½))°). = (90-2tan–¹(½))x4π÷360 = 1.287...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 22nd July, 2025

Calculating Area Red. a = (1+x) units. a is the diameter of the circle. b = ½(a) b = ½(1+x) units. b is the radius of the circle. c = ½(1-x) units. Calculating x. (½(1+x))² = 2(½(1-x))² ¼(1+2x+...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 21st July, 2025

Calculating x. Let r be the radius of the inscribed green circle. Let R be the radius of the ascribed quarter circle. (R-r)² = r²+5² --- (1). (R-r)²+5² = a² --- (2). a² = x²+r² --- (3...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 21st July, 2025

Calculating the area of the ascribed regular octagon. Let the equal longest length of the both inscribed yellow triangles be x. a = ⅛*180(8-2)) a = ⅛(180*6) a = ½(45*6) a = 135° a is the single in...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 21st July, 2025

Calculating Length AB. Let AB = (a+7+b) units. Calculating a and b. c = (7+b) units. Therefore; a(7+b) = 60 a = 60/(7+b) --- (1). Again. d = (a+7) units. Therefore; b(a+...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 21st July, 2025

Shaded Area is; Area circle with radius 4 cm - 4(area quarter circle with radius 4 cm - area isosceles right-angled triangle with equal adjacent lengths 4 cm). = π*4²-4(¼(4²π)-½(4²)) = 16π-4(4π-8...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 20th July, 2025

Calculating Red Area. A nice and careful examination of the irregular pentagon, makes a regular quadrilateral (square) with side length 1 cm. It implies; Red area is; 1² = 1*1 = 1 cm²...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 20th July, 2025

Calculating Yellow Area. Let a be the side length of the square. b = ½(a) units. c² = a²+(½(a))² c² = ¼(5a²) c = ½√(5)a units. c is the radius of the half circle. It implies; a ~ ½√(5)a d ~ a C...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 20th July, 2025

Calculating Yellow Area. a = ½(24) a = 12 units. b² = 12²+c² c = √(b²-144) units. Where; b is the ascribed half circle's radius. c is the inscribed half circle's radius. Area yellow i...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 20th July, 2025

Calculating R, radius of the circle. Notice. 3 units is the side length of the inscribed yellow square. 2 units is the side length of the inscribed red square. 1 unit is the side length of the i...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 19th July, 2025

Calculating Shaded Area. Notice. a = ½(4) a = 2 cm. a is the radius of the inscribed circle. tanb = 4/2 b = atan(2)° c = 90-b c = atan(½)° d² = 2²+4² d = √(20) cm. d = 2√(5) cm...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 19th July, 2025

Calculating x. a = 23+x+3 a = (26+x) units. b = 23-3 b = 20 units. Length x is; 21²+20² = (26+x)² 441+400 = 676+52x+x² x²+52x-165 = 0 (x+26)² = 165+26² (x+26)² = 676+165 (x+26)²...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 18th July, 2025

Area Pink is; ¼(π*3²)-½(3²)+3((¼π)-½) = ¼(9π)-½(9)+¼(3π)-½(3) = ¼(12π)-½(12) = 3π-6 = 3(π-2) square units.

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 18th July, 2025

Calculating x. Notice. AC = BC Let a be alpha. It implies; sina = 0.5x/(1+4) sina = ⅒(x) --- (1). Again. sina = 1/x --- (2). Equating (1) and (2). ⅒(x) = 1/x x² = 10 x...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 18th July, 2025

Calculating the area of the ascribed half circle. Let a be the radius of the circle. b² = a²+(3√(3))² b = √(a²+27) units. c = ½(3+b) c = ½(3+√(a²+27)) units. d = c-3 d = ½(3+√(a²+27))-3 d = ½(√(...

Mathematics Question and Solution

update

Mathematics Question and Solution

By OnlineEdumath | 17th July, 2025

Calculating red Area. Notice. 3x = 4-x 4x = 4 x = 1 unit. Where x is the radius of the red quarter circle and the inscribed white half circle. y = 4-x And x = 1 units. y = 4-1 y = 3...

Our Media

Class with Tejiri (year 12) and Vwarhe (year 10)

Class with Ellis, year 2 pupil.

Class with Ellis, year 2 pupil.

Class with Vwarhe, js3 student (year 9).

Class with Ellis, year 2 pupil.

Class with Ellis, year 2 pupil.

Class with Joshua, year 1 pupil.

Class with Ellis, year 2 pupil.

Class with Adesuwa, year 6 pupil.

Class with Prince, year 5 pupil.

Class with Ellis, year 2 pupil.

Class with Ellis, year 2 pupil.

Class with Ellis, year 2 pupil.

Mathematics and Science

Identifying Prime Numbers

Quadratic Equation Using Formula

Constructing and Bisecting Lines and Angles

Math Story

Mathematics is not difficult, it is a language

Comparing Numbers

Place Value

Telling Time

Young Sheldon

Young Sheldon

Young Sheldon

Student Bullied For Being Dumb, Turns Out He's Genius Neurosurgeon

Maths at The Mela

Math Story

The Map of Mathematics

Mathematics Knowledge

Learner's Feedback/Testimonial

Sir, my first term Mathematics result, I scored 99% and got first position

Ogheneruno

Online Edumath

Learner's Feedback/Testimonial

Sir, I was the third best pupil in class out of 30 pupils at the end of Autumn assessment

Prince

Online Edumath

Learner's Feedback/Testimonial

I was awarded Century Champion Award certificate as a celebrated Century Champion for completing my Century Work in Mathematics in my School

Oghosa

Online Edumath

Learner's Feedback/Testimonial

My Math Teacher in school do sometimes administer year 6 Math assessment to me, a year 5 pupil, and I often score 100%

Adesuwa

Online Edumath

Learner's Feedback/Testimonial

I got Bronze Certificate representing my School in the junior (year 7 and 8) Mathematics Challenge Competition, 2023 organized by United Kingdom Mathematics Trust. Oghosa is in year 7

Oghosa

Online Edumath

Learner's Feedback/Testimonial

Sir, I got A+, the highest score in my final summer Mathematics assessment score in my class.

Adesuwa

Online Edumath

Learner's Feedback/Testimonial

I scored 92% in Mathematics in my third term final Mathematics Examination assessment, Sir.

Ogheneruemu

Online Edumath

Learner's Feedback/Testimonial

Sir, I got A+, the highest score in my final summer Mathematics assessment score in my class.

Oghosa

Online Edumath

Safety and Abuse Reporting

Harassment and Cyberbullying

Impersonation

Violent Threats

Hate Speech Against a Protected Group

Spam and Scams

None of these are my issue

Thanks for being awesome!

We appreciate you contacting us. Our support will get back in touch with you soon!

Have a great day!

Are you sure you want to report abuse against this website?

Please note that your query will be processed only if we find it relevant. Rest all requests will be ignored. If you need help with the website, please login to your dashboard and connect to support