
a² = 10²+5²
a = √(125)
a = 5√(5) units.
a is the side length of the square.
b² = 2a²
b² = 2(5√(5))²
b = √(250)
b = 5√(10) units.
b is the diagonal of the square.
c = 90+atan(10/5)
c =...
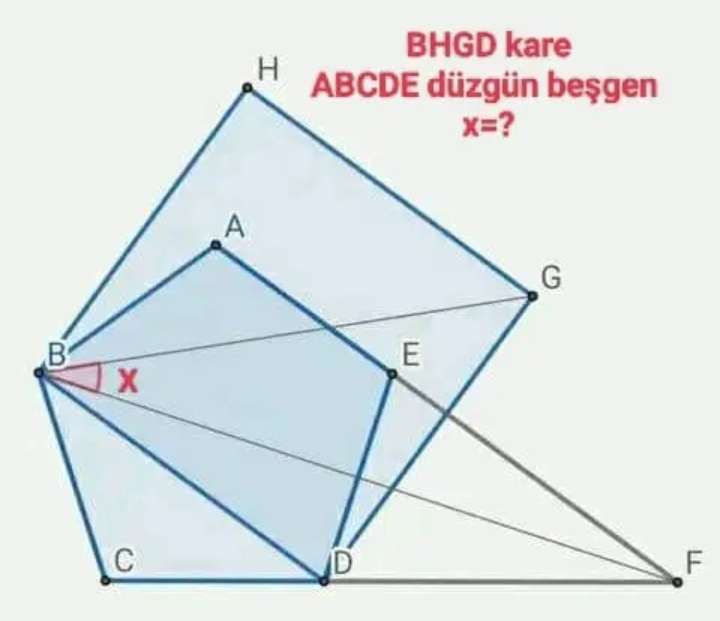
Calculating x.
a =⅕*180(5-2)
a = ⅕*180*3
a = 108°
a is the single interior angle of the regular pentagon.
b = ½(180-108)
b = ½(72)
b = 36°
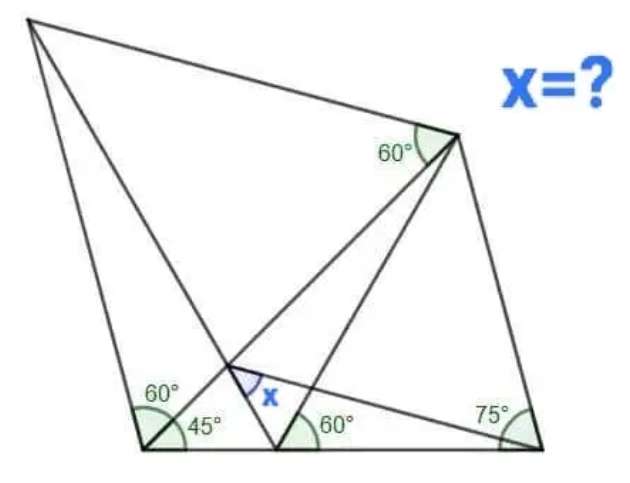
c = ½(180-2(180-108))
c = ½(180-144)
c = ½...
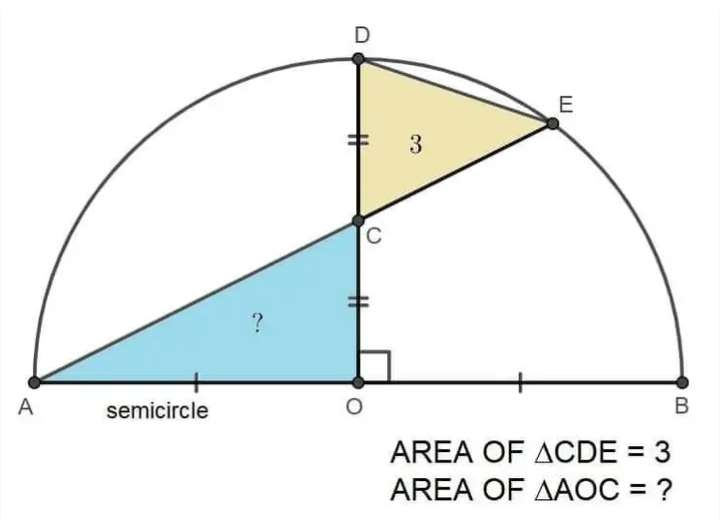
Let a be the radius of the ascribed half circle.
tanb = a/(0.5a)
b = atan(2)°
b is angle OCA = angle DCE.
c = atan(½)°
c is angle OAC.
d² = a²+(½(a))²
d = √(¼(5a²))
d = ½√(5)a² units....
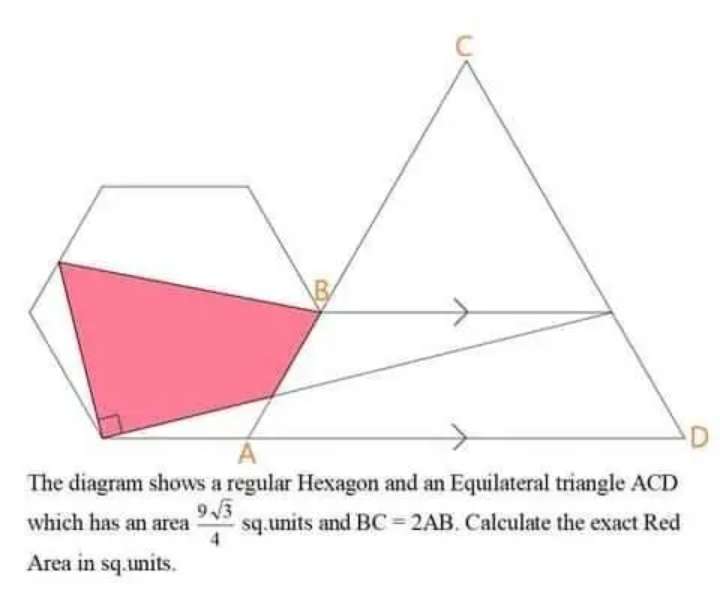
Area red in its exact simplest decimal square unit form is;
Area triangle with height and base 1.24899959968 units and 1.20185042515 units + Area triangle with side ⅔ units and (26/15) units, an...
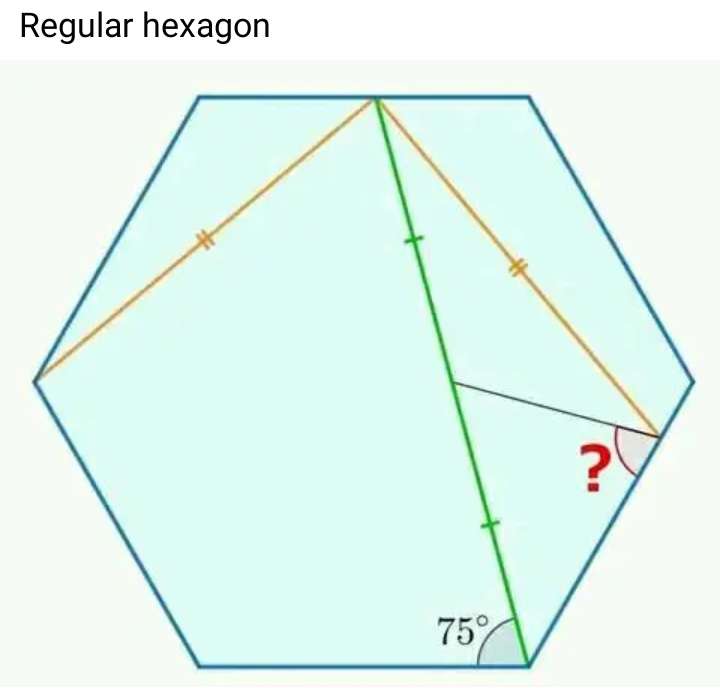
Let the height of the regular hexagon be 1 unit.
tan75 = 1/a
a = 0.2679491924 units.
sin75 = 1/b
b = 1.0352761804 units.
c = ½(b)
c = 0.5176380902 units.
1 = 2d²-2d²cos120
d is the...
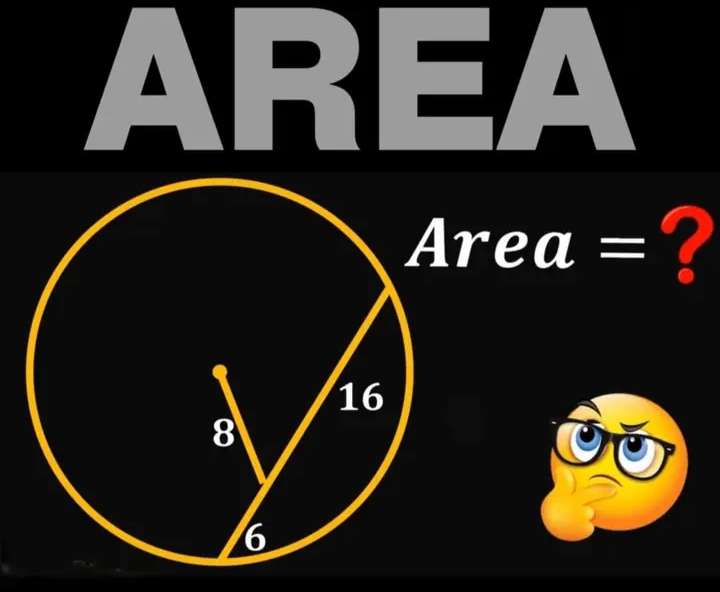
Let a be the radius of the circle.
a² = 6²+8²+2*6*8cosb
a² = 100+96cosb --- (1).
a² = 16²+8²-2*16*8cosb
a² = 320-256cosb --- (2).
Equating (1) and (2).
100+96cosb = 320-256cosb
cosb...
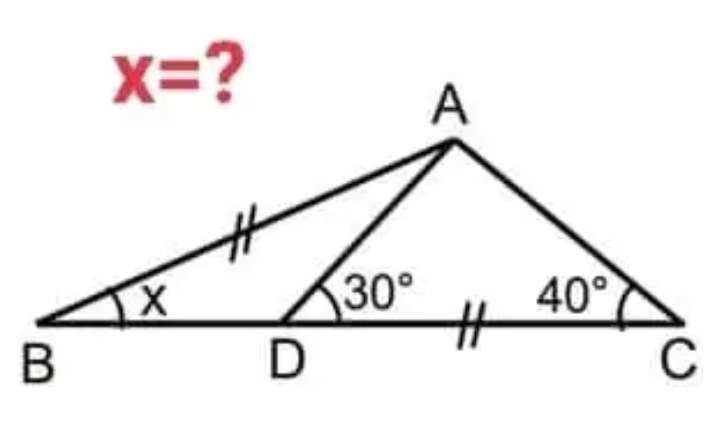
Let CD = AB = 1 unit.
a = 180-30-40
a = 110°
a is angle CAD.
(1/sin110) = (b/sin30)
b = 0.5320888862 units.
b is AC.
Therefore, the required angle, x is;
(0.5320888862/sinx) = (1/si...
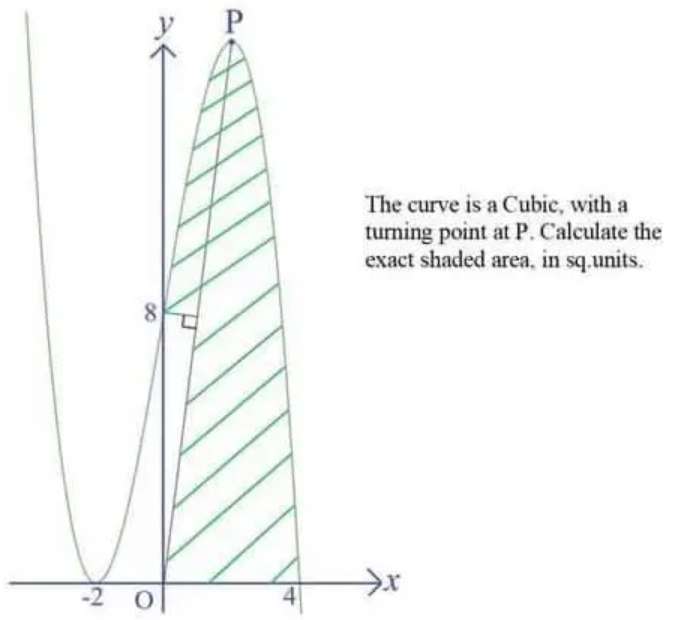
Sir Mike Ambrose is the author of the question.
The equation of the curve is;
y = -½(x+2)²(x-4)
y = -½(x²+4x+4)(x-4)
y = -½(x³-12x-16)
y = -½(x³)+6x+8
It implies;
dy/dx = -½(3x²)+6
Fo...
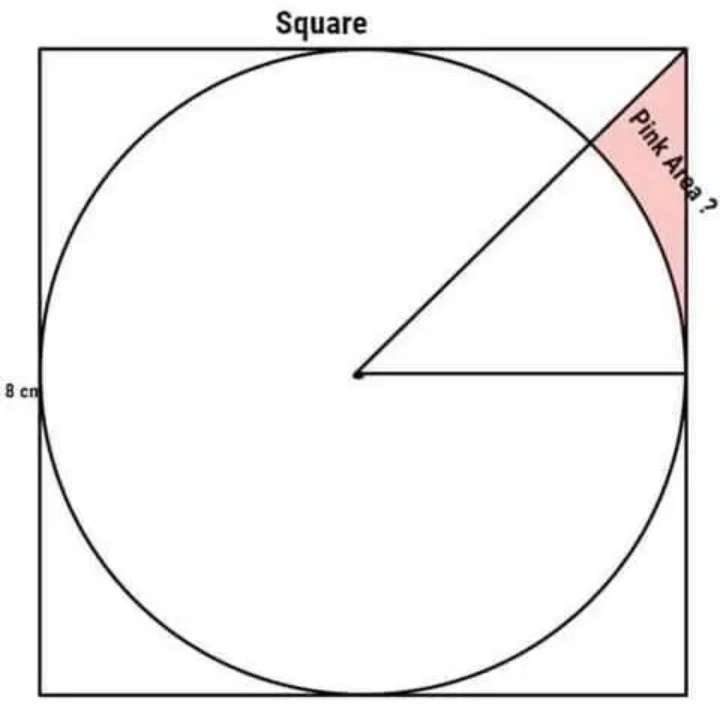
Pink area is;
½(area square with side 4 cm - area quarter circle with radius 4 cm)
= ½(4*4 - ¼(16π))
= ½(16 - 4π)
= 8 - 2π
= 2(4-π) cm²