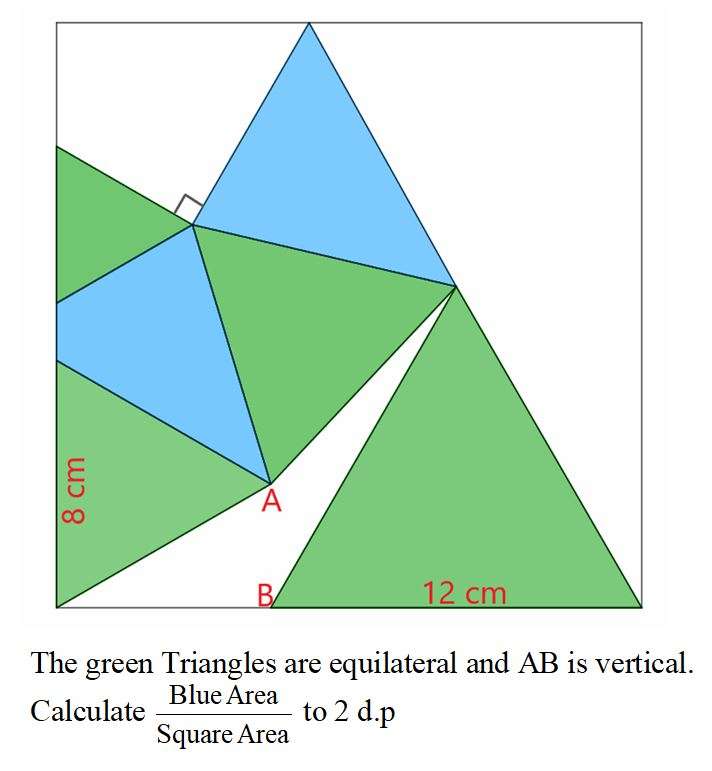
Mathematics Question and Solution
Let a be the side of the square.
cos30 = b/8
b = 4√(3) cm.
sin30 = c/8
c = 4 cm.
a = b+12
a = (12+4√(3)) cm.
Area Square is;
a²
= (12+4√(3))²
= 358.27687752661 cm²
d² = 12²+4²-2*4*12cos30
d = 8.76707255797 cm.
(8.76707255797/sin30) = (12/sine)
e = 180-43.18678543201
e = 136.81321456799°
f = 360-e-180
f = 43.18678543201°
g² = 8²+8.76707255797²+2*8*8.76707255797cos43.18678543201
g = 6.21165708246 cm.
(6.21165708246/sin43.18678543201) = (8.76707255797/sinh)
h = 75°
i = 120-757.54700538379
i = 45°
(j/sin45) = (6.21165708246/sin120)
j = 5.07179676972 cm.
Where j is the side length of the smallest green equilateral triangle.
k = 180-120+45
k = 15°
(l/sin15) = (5.07179676972/sin45)
l = 1.85640646055 cm.
m = (12+4√(3))-8-5.07179676972-1.85640646055
m = 4 cm.
n² = 4²+5.07179676972²-2*4*5.07179676972cos120
n = 7.87466250402 cm.
(4/sino) = (7.87466250402/sin120/
o = 26.09780538934°
p = 90-26.09780538934
p = 63.90219461066°
q = 60-26.09780538934
q = 33.90219461066°
r = 90-33.90219461066
r = 56.09780538934°
s = 180-56.09780538934-63.90219461066
s = 60⁰
(7.87466250402/sin60) = (t/sin56.09780538934)
t = 7.54700538379 cm.
(8.76707255797/sin75) = (8/sinu)
u = 61.81321456797°
v = 150-15-61.81321456797
v = 73.18678543203°
Area blue is;
0.5*7.54700538379*8.76707255797sin73.18678543203 + 0.5*8*6.21165708246sin75 + 0.5*6.21165708246*5.07179676972sin15
= 31.66838492538 + 24 + 4.07695154586
= 59.74533647124 cm²
Area Blue ÷ Area Square to 2 decimal places is;
59.74533647124 ÷ 358.27687752661
= 0.16675744436
≈ 0.17