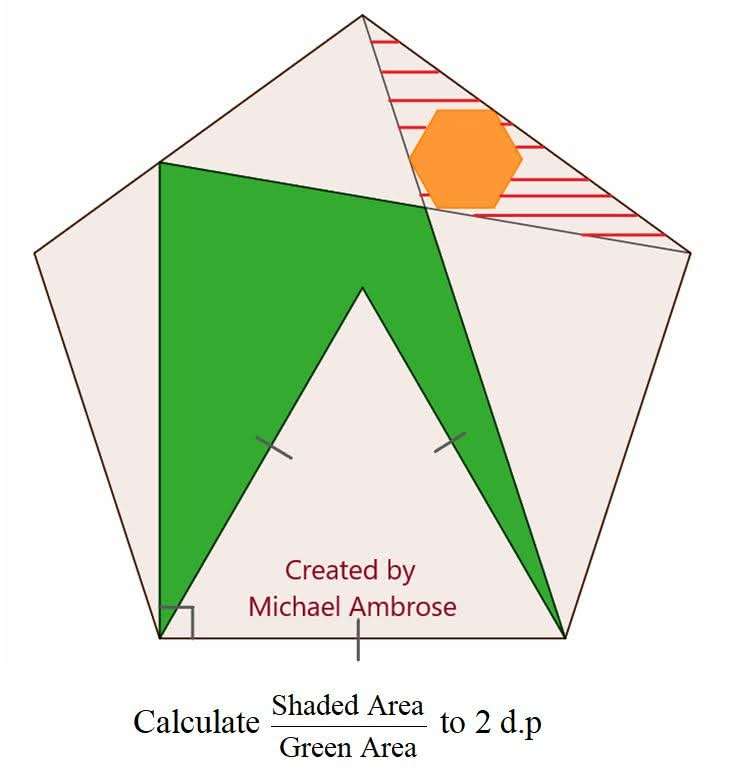
Mathematics Question and Solution
Let the side of the regular pentagon be 1 unit.
a = 108-90
a = 18°
b = 180-108-18
b = 54°
(1/sin54) = (c/sin108)
c = 1.17557050458 units.
(1/sin54) = (d/sin18)
d = 0.38196601125 units.
e = 1-d
e = 0.61803398875 units.
f² = 0.61803398875²+1²-2*0.61803398875cos108
f = 1.3281310261 units.
(1.3281310261/sin108) = (1/sing)
g = 45.73230144788°
h = 180-108-45.73230144788
h = 26.26769855212°
I = 180-72-45.73230144788
i = 62.26769855212°
(0.61803398875/sin62.26769855212) = (j/sin72)
j = 0.66406551305 units.
k = 180-36-26.26769855212
k = 117.73230144788°
l² = 2-2cos108
l = 1.61803398875 units.
(0.66406551305/sin72) = (m/sin45.73230144788)
m = 0.5 units.
n = 1.61803398875-m
n = 1.11803398875 units.
Area Green is;
0.5*1*1.17557050458+0.5*0.66406551305*1.11803398875sin117.73230144788-0.5sin60
= 0.48335449547 square units.
Let a circle ascribe the inscribed orange regular hexagon with the circle's circumference touching the 6 vertices of the orange regular hexagon.
Let o be the radius of the now inscribed circle.
Calculating o.
(o/tan18)+(o/tan(0.5*26.26769855212)) = 1
o = 0.13580578978 units.
p = 2o
p = 0.27161157957 units.
Let the side of the regular hexagon be q.
Calculating q.
sin30 = r/q
r = qsin30
It implies;
q+2qsin30 = 0.27161157957
q = 0.27161157957/(1+2sin30)
q = 0.13580578978 units.
Area Shaded is;
0.5*1*0.5sin36-0.5*0.13580578978*0.13580578978*6(1/(2tan30))
= 0.09902944132 square units.
Shaded Area ÷ Area Green to 2 decimal places is;
0.09902944132÷0.48335449547
= 0.20487952889
≈ 0.20